The friction at sleeve A can provide a maximum resisting moment of 125 N•m about the x axis. Determine the largest magnitude of force F that can be applied to the bracket so that the bracket will not turn.

 The friction at sleeve A can provide a maximum resisting moment of 125 N•m about the x axis. Determine the largest magnitude of force F that can be applied to the bracket so that the bracket will not turn.

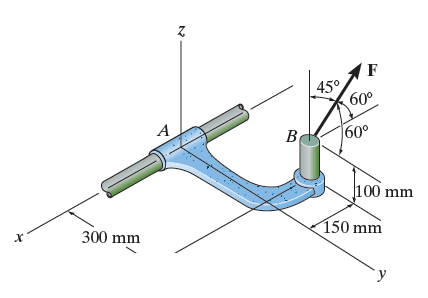
Image from: Hibbeler, R. C., S. C. Fan, Kai Beng. Yap, and Peter Schiavone. Statics: Mechanics for Engineers. Singapore: Pearson, 2013.

Solution:

Let us first express force F in Cartesian form. (Don’t remember?)

$F=\left\{-F\cos60^0i+F\cos60^0j+F\cos45^0k\right\}$

(simplify)

$F=\left\{-0.5F\,i+0.5F\,j+0.707F\,k\right\}$

 

We will now draw a position vector from A to B as follows:

Let us now calculate $r_{AB}$:

$r_{AB}=\left\{(-0.15-0)i+(0.3-0)j+(0.1-0)k\right\}$

$r_{AB}=\left\{-0.15i+0.3j+0.1k\right\}$

 

We can now calculate the moment along the x-axis. Remember that the unit vector for the x-axis is i.

$M_x=i\cdot r_{AB}\times F$

$M_x=\begin{bmatrix}1&0&0\\-0.15&0.3&0.1\\-0.5F&0.5F&0.707F\end{bmatrix}$

Taking the cross product gives us:

$M_x=0.1621F$

 

Substitute the maximum resisting moment (given to us in the question):

$125=0.1621F$

$F=771$ N