The cantilevered beam AB is additionally supported using a tire rod BD. By using Virtual Work Method deterkine the force in the rodand draw the bending moment diagram.Neglect effect of axial compression and shear in the beam.for the beam I =200(10^6)mm^4

 

Use the moment distribution method to determine the end moments of the members of the frame given below and draw the bending moment diagram

  

Using the slope deflection Method, adetermine the reaction and draw the bending moment diagram for the structure shown below.

 

Draw the Shear and Moment diagrams for the beam. Indicate values at the supports and at the points where a change in load occurs.

 Draw the Shear and Moment diagrams for the beam. Indicate values at the supports and at the points where a change in load occurs.

Solution:

Determine the internal shear and moment in the beam as a function of x.

 Determine the internal shear and moment in the beam as a function of x.

Solution:

Using the method of joints,Determine the axial force in each member of Truss shown in figure.

Using the method of joints,Determine the axial force in each member of Truss shown in figure.

 

Draw the Shear and Moment Diagram for the beam. Indicate values at the supports and at points where change in load occurs.

 Draw the Shear and Moment Diagram for the beam. Indicate values at the supports and at points where change in load occurs.

Solution:

Let RA and RB are the vertical support reactions at A and B, and MA and MB be the bending moments at the same.

As per the Conditions of Static Equilibrium of the Structural Element:
∑Fy = 0, ∑Fx = 0, and ∑M = 0

Values at Supports:

The Shear Force at the left support A, VA = 0.5*[(-4.0)] * 1.5
or VA = -3.0 kN

The bending moment at the left support A, MA =0.5 * (-4.0) * (1.5) * ( 1.5/3)

Hence, MA = -1.5 kNm

The Shear Force at the right support B, VB = 0.5*[(4.0)] * 1.5
or VB = 3.0 kN

The bending moment at the right support B, MB = 0.5 * (-4.0) * (1.5) * (1.5/3)

Hence, MB = -1.5 kNm

Using the static equilibrium conditions,
∑Fy = 0, RA + RB = 0.5 * [4.0] * (1.5) + 4 * (2.0) + 0.5 * [4.0] * (1.5)

Hence RA + RB = 14.0 kN — eq(1)
Also, ∑M =0, hence the algebraic sum of the moments at left support is equated to zero.
Hence, RB*(5.0-1.5-1.5) = [(-1.5) – (-1.5) + (3.0)(5.0 – 1.5 – 1.5) -4.0 * (2.0) * 1.0

Hence, RB = 7.0 kN
From eq(1), RA = 7.0 kN

Shear and Moment equations:

In between A and B,
for 1.5m <= x <= 3.5m:
Shear, Vx = 10.0 – 4.0*x

Moment, Mx = VA(x-1.5) + -4.0(x -1.5)^2/2 = -2.0x2 + 10.0x – 12.0

In between B and the right end,
for 3.5m <= x <= 5.0m:
Load intensity at x, wx = 2.667x – 13.33

Shear, Vx = 1.333x*2 – 13.33x + 33.33

Moment, Mx = 0.445x3 – 6.667x2 + 33.33x – 55.55

Shear Force and Bending Moment Diagram:

distance x. Shear V Moment M
0.0. 0.0. 0.0
1.5 4.0 -1.5
3.5 3.0. -1.5
5.0 0.0 0.0

Maximum +ve Shear Force, Vmax = 4.0 kN
at x = 1.5 m from the left end.

Maximum -ve Shear Force, Vmin = -4.0 kN
at x = 3.5 m from the left end.

Maximum +ve Bending Moment, Mmax = 0.5 kN-m
at x = 2.5 m from the left end.

Maximum -ve Bending Moment, Mmin = -1.5 kN-m
at x = 3.5 m from the left end.

Determine the internal normal force, shear force and bending moment acting at point C in the beam shown in the figure.

 Determine the internal normal force, shear force and bending moment acting at point C in the beam shown in the figure.

Reference: Structural Analysis by R C Hibbeler.

Solutio

L = 4 m,n:

VB = 0.5*5*2 = 5 kN

please note that at supports, MA = 0.0 kNm, and MB = -0.5*5*2*2/3 = -3.334 kN m

Using the static equilibrium conditions, algebraic sum of moments at the left end is equated to zero.
Hence, RB = [(MB – MA – VB*(L -2) -5.0 * (2.0) * 1.0 ] / (L-2.0)
Hence, RB = 11.667 kN
Also, RA + RB = 5*2 + VB = 15 kN.
Solving gives, RA = 3.334 kN,

End support reactions are: Vertical Reactions, RA = 3.334 kN, and RB = 11.664 kN

In between A and B,
for 0.0m <= x <= 2.0m:
Shear, Vx = 3.334 – 5.0*x

Moment, Mx = VA(x-0.0) + -5.0(x -0.0)^2/2 = -2.5x^2 + 3.334*x

In between B and the right end,

for 2.0m <= x <= 4.0m:
Shear, Vx = 1.25x2 – 10.0x + 20.0

Moment, Mx = 0.41667x^3 – 5.0*x^2 + 20.0*x – 26.667

Shear Force and Bending Moment Diagram, F4-4, Structural Analysis by R C Hibbeler.

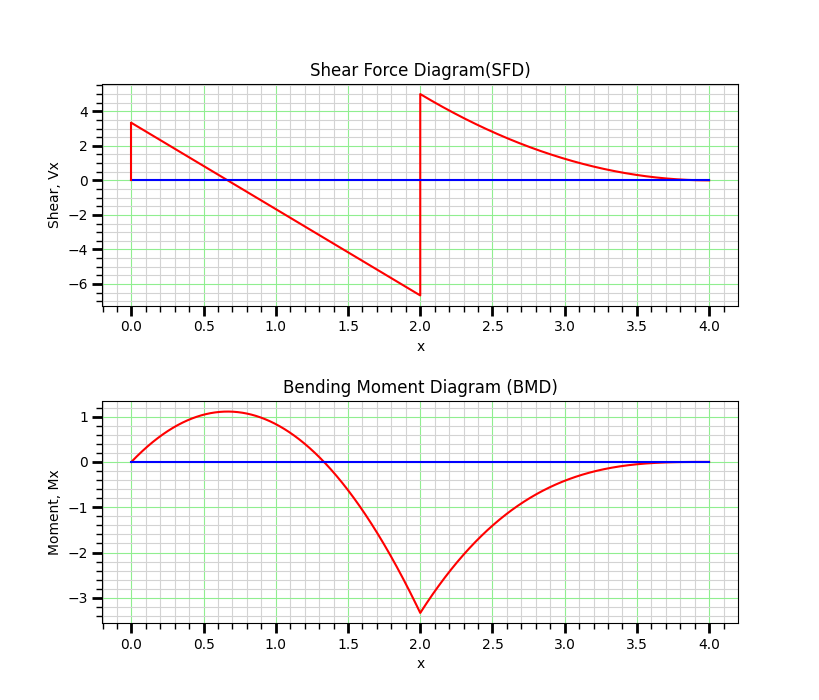
Maximum +ve Bending Moment, Mmax = 1.111 kN-m
at x = 0.667 m from the left end.

Maximum -ve Bending Moment, Mmin = -3.333 kN-m
at x = 2.0 m from the left end.

At C, x = 1 m,

Axial Force, NC = 0,

Shear Force, VC = 3.334 – 5.0*1 = -1.6667 kN

Bending Moment, MC = -2.5* 12 + 3.334*1 = 0.8334 kN-m

A uniform beam has a mass of 18 kg and rests on two surfaces at points A and B. Determine the maximum distance x to which the girl can slowly walk up the beam before it begins to slip. The girl has a mass of 50 kg and walks up the beam with a constant velocity.

 A uniform beam has a mass of 18 kg and rests on two surfaces at points A and B. Determine the maximum distance x to which the girl can slowly walk up the beam before it begins to slip. The girl has a mass of 50 kg and walks up the beam with a constant velocity.

The solution is;

Let θ be the internal angle at B
sinθ = 1.8/3.0
sinθ = 0.6
θ = 36.87.

At B, the normal force of the floor on the ramp Nb is vertical.
At A, the normal force of the step on the ramp Na is perpendicular to the ramp or θ from vertical.

Sum horizontal forces to zero. Assume right is the positive direction.

Na(sinθ) - μ(Na)cosθ - μNb = 0.
Na(sinθ - μcosθ) = μNb.
Nb = Na(0.6 - 0.2(0.8)/0.2.
Nb = 2.2Na.

Sum vertical forces to zero. Assume up is positive.

Nb + Na(cosθ) + μ(Na)sinθ - (M + m)g = 0.
2.2Na + Na(cosθ) + μ(Na)sinθ - (M + m)g = 0,
Na (2.2 + cosθ + μsinθ) = (M + m)g,
Na = (M + m)g / (2.2 + cosθ + μsinθ),
Na = (50 + 18)9.81 / (2.2 + 0.8 + 0.2(0.6)),
Na = 213.8.

Sum moments about B to zero. Assume clockwise moment is positive'.

Nb and the friction force at A have no moment arm so create no moment about B.
Na[3.0] - Mg[xcosθ] - mg[(3.5 / 2)cosθ] = 0.

x = (3Na - mg[1.75cosθ])/Mgcosθ.
x = (3(213.8) - 18(9.81)1.75(0.8))/50(9.81)0.8.
x = 1.005 m.

The five-story office steel building shown in Figure 2.7 is laterally braced with steel special moment resisting frames, and it measures 75 ft by 100 ft in the plan. The building is located in New York City. Using the ASCE 7-16 equivalent lateral force procedure, determine the lateral force that will be applied to the fourth floor of the structure. The roof dead load is 32 psf, the floor dead load (including the partition load) is 80 psf, and the flat roof snow load is 40 psf. Ignore the weight of cladding. The design spectral acceleration parameters are SDS = 0.28, and SD1 = 0.11.

 The five-story office steel building shown in Figure 2.7 is laterally braced with steel special moment resisting frames, and it measures 75 ft by 100 ft in the plan. The building is located in New York City. Using the ASCE 7-16 equivalent lateral force procedure, determine the lateral force that will be applied to the fourth floor of the structure. The roof dead load is 32 psf, the floor dead load (including the partition load) is 80 psf, and the flat roof snow load is 40 psf. Ignore the weight of cladding. The design spectral acceleration parameters are SDS = 0.28, and SD1 = 0.11.

Fig. 2.7. Five – story office building.

Solution

SDS = 0.28 and SD1 = 0.11 (given).

R = 8 for special moment resisting steel frame (see Table 2.13).

An office building is in occupancy risk category II, so Ie = 1.0 (see Table 2.9).

Calculate the approximate fundamental natural period of the building Ta.

Ct = 0.028 and x = 0.8 (from Table 2.12 for steel moment resisting frames).

ℎn = Roof height = 52.5 ft

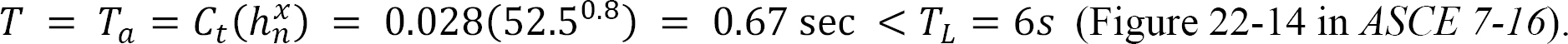
Determine the dead load at each level. Since the flat roof snow load given for the office building is greater than 30 psf, 20% of the snow load must be included in the seismic dead load computations.

The weight assigned to the roof level is as follows:

Wroof = (32 psf)(75 ft)(100 ft) + (20%)(40psf)(75 ft)(100 ft) = 300,000 lb

The weight assigned to all other levels is as follows:

Wi = (80 psf)(75ft)(100 ft) = 600,000 lb

The total dead load is as follows:

WTotal = 300,000 lb + (4)(600,000 lb) = 2700 k

Calculate the seismic response coefficient Cs.

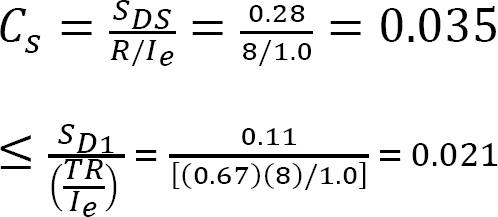
Therefore, Cs = 0.021 > 0.01

Determine the seismic base shear V.

V = CsW = (0.021)(2700 kips) = 56.7k

Calculate the lateral force applied to the fourth floor.

2.1.4.5 Hydrostatic and Earth Pressures

The reaction at support A of the beam shown in below figure, is

The reaction at support A of the beam shown in below figure, is

 Solution

 Simply moment at pin and roller support is zero, but if you want to how to get its value.

Ray+Rby=1*10,Ray+Rby=10.
Then, take moment at support a,Ma =0,Rby*10-10*5=0, Rby=5
Then, Ray+Rby=10,Ray=5.
Take moment at A,Ma=10*5-Rby * 10,
Ma=10*5-5*10,
Ma=0.

The principles of a differential chain block are indicated schematically in the figure. Determine the magnitude of force P needed to support the 800-N force. Also compute the distance x where the cable must be attached to bar AB so the bar remains horizontal. All pulleys have a radius of 60 mm

The principles of a differential chain block are indicated schematically in the figure. Determine the magnitude of force P needed to support the 800-N force. Also compute the distance x where the cable must be attached to bar AB so the bar remains horizontal. All pulleys have a radius of 60 mm

Solution

 At Left block.

Each rope on left block 800/4=200N.
Last rope also is equal 200N.

At Right block:
Same principle,
P=200/5=40N.

Let's call left block top line joint points A, B(hook point), C, D.
B=200.
Each Pulley point load is 40+40=80N.
C=80.
D=80.
sum of M(A)=0.
200 * x - 180 * 80 - 420 * 80 = 0.
200 * x = 48000.
x = 240mm.

Determine the force in each member of the truss and indicate whether the members are in tension or compression.

Determine the force in each member of the truss and indicate whether the members are in tension or compression.

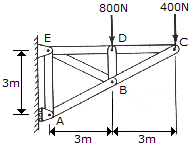
Solution

First consider a free body diagram for the whole truss structure to find the reactions at supports E and A by applying the equations of equilibrium for its external forces.

So taking summation of moment about E = 0, 3Ra-3(800)-6(400) = 0.

Ra = 1600 N.

Sum of horizontal forces = Re(x)-800-400 = 0; Re(x) = 1200 N.

Sum of vertical forces = Re(y)-Ra = 0; Re(y) = 1600 N.

Considering each member force to be a tension, each joint will be analyzed so taking joint C,

Sum of horizontal forces = 400+F(CB) sin(26.57) = 0, F(CB) = -894 N T or F(CB) = 894 N C.

Sum of vertical forces = -F(CB) cos (26.57)-F(CD) = 0; F(CD) = 800 N T.

At joint D, F(DC) = F(DE) = 800 N T F(DB) = -800 N T = 800 N C.

At joint E, Sum of vertical forces = F ED)+F(EB) sin(63.43)-Re(y) = 0.

F(EB) = (Re(Y)-F(ED))/sin(63.43) = 894 N T.

Sum of horizontal forces = F(EA) = F(EB) cos(63.43) = Re(x).

F(EA) = 1200-894 cos(63.43) = 800 N T.

At joint B, Sum of horizontal forces = 0.

F(BA) cos(63.43)-F(BC) cos(63.43)-F(BE) cos(63.43)-F(BD) = 0.

F (BA) = -1789 N T = 1789 N C.

Determine the force in each member of the Fink truss shown in Fig.

 Determine the force in each member of the Fink truss shown in Fig.

Solution
The Fink truss shown in Fig.  is a compound truss formed by connecting two simple trusses, ACL and DFL, by a common joint L and a member CD.

Symmetry Since the geometry of the truss and the applied loading are symmetrical about the center line of the truss (shown in Fig. )), its member forces will also be symmetrical with respect to the line of symmetry. It is, therefore, su‰cient to determine member forces in only one-half of the truss. The member forces determined here for the left half of the truss are shown in Fi). The forces in the right half can be obtained from the consideration of symmetry; for example, the force in member MN is equal to that in member JK, and so forth. The reader is urged to verify this by computing a few member forces in the right half of the truss.

Determine the force in each member of the compound truss shown in Fig.

 Determine the force in each member of the compound truss shown in Fig.

Solution

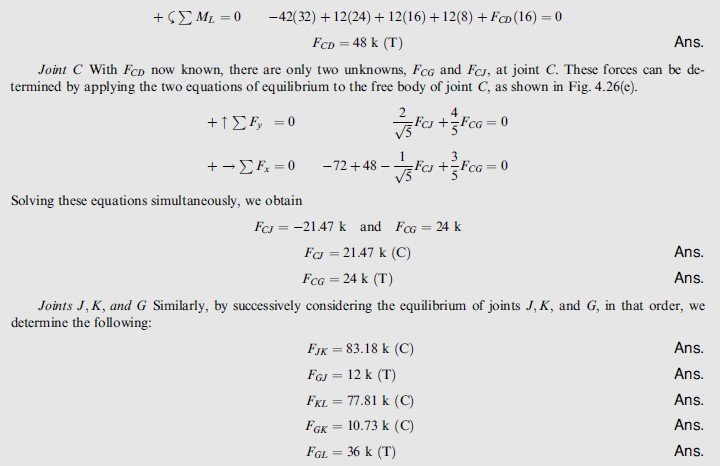
Static Determinacy The truss has 11 members and 7 joints and is supported by 3 reactions. Since m þ r ¼ 2j and the reactions and the members of the truss are properly arranged, it is statically determinate. The slopes of the inclined members, as determined from the dimensions of the truss, are shown in Fig. 4.25(a). Reactions The reactions at supports A and B, as computed by applying the three equilibrium equations to the freebody diagram of the entire truss  are

With FAB now known, the method of joints can be started either at joint A, or at joint B, since both of these joints have only two unknowns each. We begin with joint A.
Joint A The free-body diagram of joint A is shown in Fig. 4.25(d).

Compute the midspan value of EIδ for the beam shown in Fig. . (Hint: Draw the M diagram by parts, starting from midspan toward the ends. Also take advantage of symmetry.

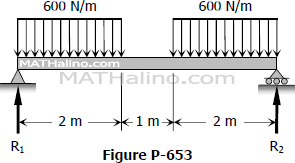
Compute the midspan value of EIδ for the beam shown in Fig. . (Hint: Draw the M diagram by parts, starting from midspan toward the ends. Also take advantage of symmetry.
 

Solution (Using Moment Diagram by Parts)

 By symmetry,

R1=R2=2(600)$R_1=R_2=2(600)$

R1=R2=1200 N$R_1=R_2=1200\text{ N}$
 

 

 

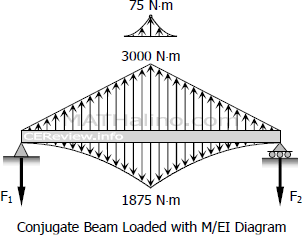
The loads of conjugate beam are symmetrical, thus,
F1=F2=12[12(5)(3000)+13(1)(75)−13(5)(1875)]$F_1=F_2=\frac{1}{2}[\frac{1}{2}(5)(3000)+\frac{1}{3}(1)(75)-\frac{1}{3}(5)(1875)]$

F1=F2=2200 N⋅m2$F_1=F_2=2200\text{ N}\cdot {\text{m}}^2$
 

For this beam, the maximum deflection will occur at the midspan.
 

 

Mmidspan=12(2.5)(3000)[13(2.5)]+13(0.5)(75)[14(0.5)]−13(2.5)(1875)[14(2.5)]−2200(2.5)$M_{midspan}=\frac{1}{2}(2.5)(3000)[\frac{1}{3}(2.5)]+\frac{1}{3}(0.5)(75)[\frac{1}{4}(0.5)]-\frac{1}{3}(2.5)(1875)[\frac{1}{4}(2.5)]-2200(2.5)$

Mmidspan=−3350 N⋅m3$M_{midspan}=-3350\text{ N}\cdot {\text{m}}^3$
 

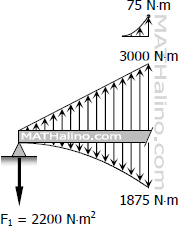
Therefore, the maximum deflection is
EI δmax=Mmidspan$EI{\delta }_{max}=M_{midspan}$

EI δmax=−3350 N⋅m3$EI{\delta }_{max}=-3350\text{ N}\cdot {\text{m}}^3$

EI δmax=3350 N⋅m3$EI{\delta }_{max}=3350\text{ N}\cdot {\text{m}}^3$   below the neutral axis           answer